SEQUENCE & SERIES

Sequence and Series

Arithmetic Progression (AP)

• An arithmetic progression is a sequence of numbers in which each term after the first is obtained by adding a constant ‘d’ to the preceding term. The constant d is called the common difference.

 

• An arithmetic progression is given by a, (a + d), (a + 2d), (a + 3d), …

where a = the first term, d = the common difference

 

• If a, b, c are in AP then b = (a + c)/2
• n^th term of an arithmetic progression

t_n = a + (n – 1)d

where t_n = n^th term, a= the first term, d= common difference

 

• Number of terms of an arithmetic progression

n=(l-a)/d+1

where n = number of terms, a= the first term , l = last term, d= common difference

 

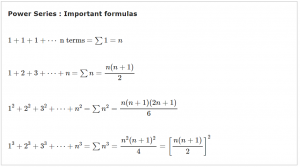
FORMULAS

ADDITIONAL NOTES ON AP

To solve most of the problems related to AP, the terms can be conveniently taken as

3 terms: (a – d), a, (a +d)

4 terms: (a – 3d), (a – d), (a + d), (a +3d)

5 terms: (a – 2d), (a – d), a, (a + d), (a +2d)

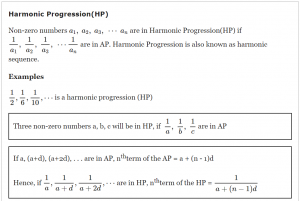
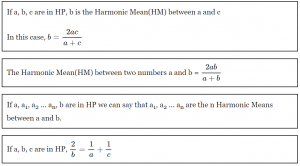
Harmonic Progression(HP)

Geometric Progression (GP)

Geometric Progression (GP) is a sequence of non-zero numbers in which the ratio of any term and its preceding term is always constant.